Chapter 1: How Science Works

An image showing the commutativity of addition

The system for manipulating numbers and symbols in an equation is called algebra. Scientists use equations to express physical relationships between measurable quantities. Algebra is the tool that scientists use to relate one equation to another, or to convert an equation into a more useful form. In algebra, we use letters of the alphabet (a,b,c…x,y,z) as symbols to represent numbers or physical quantities. The idea is to manipulate the symbols without inserting numbers until you have the equation in the form you want.

 

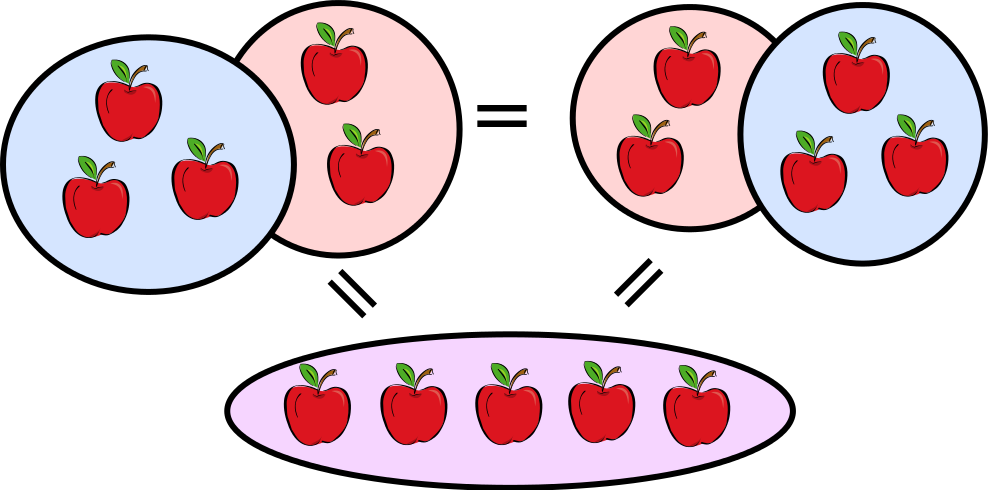
Algebra has simple rules that are designed to let you manipulate an equation to get the quantity you want on the left-hand side of the equal sign. The equations you will see in basic astronomy are simple, but the same rules can be used to solve equations of enormous complexity. In algebra, whatever you do to one side of an equation, you must also do to the other side. For example, if x = y, then x/2 = y/2 is true and x-7 = y-7 is true. The rule applies for any operation, so x³ = y³ is true, and log x = log y is true. An equality is always true as long as the same operation is performed on both sides of the equation.

• Addition and Subtraction: If this is true, a+b = c, then this is true, a = c-b

• Multiplication and Division: If this is true, a/b = c/d, then this is true, ad = bc

• Powers and Roots: If this is true, a = b², then this is true, √a = b

The rules of algebra tell us how to manipulate an equation to get the result we want. To "solve" an equation for a certain quantity means removing all other numbers and symbols so that the equation has only the quantity we want on the left-hand side. If the unwanted number or symbol is added, we remove it by subtracting it from both sides of the equation. In the example from the table above, a+b=c, we solve for a by subtracting b from both sides of the equation, giving a+b-b=c-b, or a=c-b. In general, we always perform the inverse operation — remove a quantity that has been multiplied by dividing it, remove a quantity that has been subtracted by adding it, and so on.

Thermometer with Fahrenheit and Celsius units. We can use algebra to convert one temperature unit to the other.

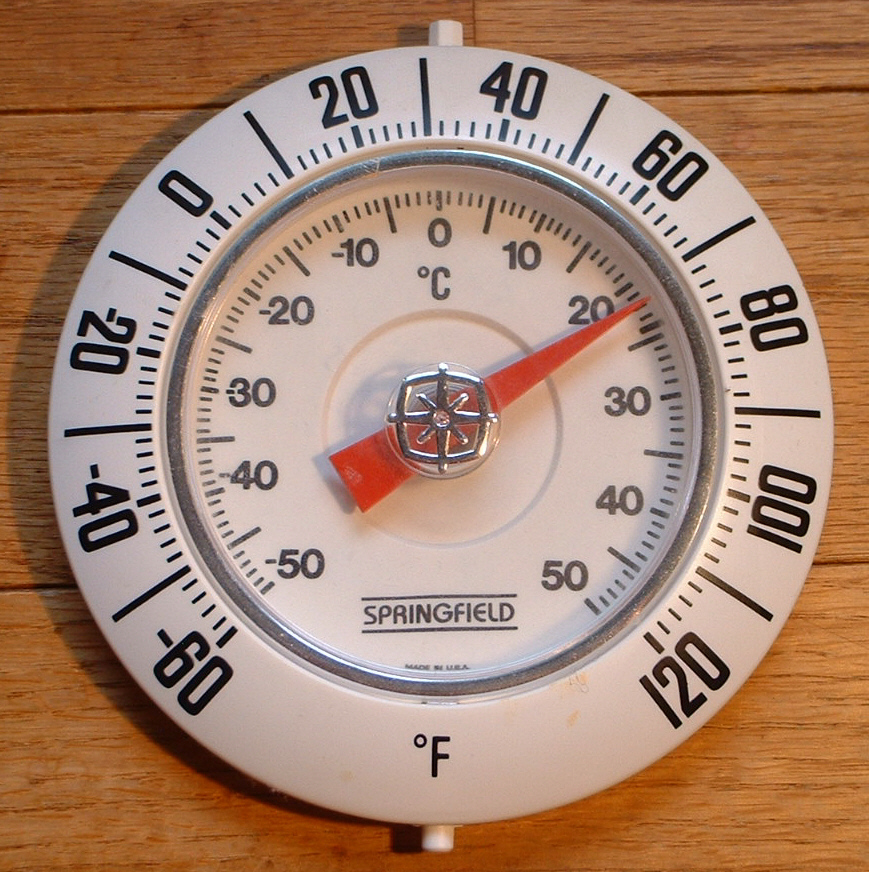
Suppose you want to convert a temperature in the Fahrenheit system into the Celsius system (or degrees centigrade). The equation that relates the two is C = 5/9(F-32). You need to solve this equation for F; that is, you need to get the quantity F alone on the left-hand side of the equation. First, multiply each side by 9, giving 9C = 9×5/9(F-32). The nines on the right-hand side cancel to give 9C = 5(F-32). Now divide each side by 5, giving 9C/5 = 5/5(F-32). The fives on the right-hand side cancel to give 9C/5 = F-32. Next, add 32 to each side, giving 9C/5+32 = F-32+32. The result is 9C/5+32 = F and we can just reverse the equation to get the form we want, F = 9C/5+32.

 

Newton's law gives the force in terms of the mass acted on and the amount of acceleration caused, F = ma. Suppose we want to solve this equation for the acceleration. Simply divide each side of the equation by mass, F/m = ma/m. The masses on the right-hand side cancel, giving the result F/m = a, or a = F/m. Einstein's famous equation that gives the energy locked up in matter is E = mc². To find the mass equivalent to a certain amount of energy, we need to get the mass on its own, so divide each side of the equation by c². The result is E/c² = m, or m = E/c².

Finally, the kinetic energy of a moving object is given by the equation E = ½ mv². Suppose we know the energy and mass of the object and want to solve for its velocity. First, we multiply both sides of the equation by two, giving 2E = mv². Then we divide both sides by m, giving 2E/m = v². Finally, we take the square root of both sides of the equation, giving √(2E/m) = v. So we have our answer, the velocity in terms of the kinetic energy and mass is v = √(2E/m).

The equations in astronomy have a variety of simple forms. Remember that these equations are not just abstract pieces of mathematics — each equation expresses a relationship between quantities that we can measure in the real world. Equations are the tools used by scientists to help make sense of the physical universe.

The simplest form of equation is a direct relationship between two quantities, which we can write as:

y = kx

In this equation, x and y are two quantities we can measure and k is a number, or constant. In the everyday world, y might be the cost of something and x might be its weight. The constant of proportionality, k, is the price per pound, or the price per kilogram. We can also write:

y ∝ x

In this form, the symbol ∝ means "proportional to" and we say that y is directly proportional to x. In other words, if the weight doubles, the cost doubles, and if the weight triples, so does the cost.

There is another useful form of a direct relationship that comes from taking two specific situations. In one case, y₁ = kx₁, and in the second case, y₂ = kx₂. If we divide the two equations, the constant of proportionality cancels out and we get the result:

y₁/y₂ = x₁/x₂

In other words, the ratio of the costs of two items is equal to the ratio of their weights (assuming that k is the same for each item). To take another example, F = ma, this means that when an object is subject to a force, the acceleration is proportional to the force. If you double the force, the acceleration will double too.

The list below shows the main types of relationships between quantities that you will encounter in your study of science.

• Direct: y ∝ x, Example, E ∝ m (Mass-Energy)

• Inverse: y ∝ 1/x, Example, l ∝ 1/T (Wien's law)

• Square: y ∝ x², Example, E ∝ v² (Kinetic energy)

• Inverse square: y ∝ 1/x², Example, F ∝ 1/r² (Newton's gravity)

• Cubic: y ∝ x³, Example, V ∝ r³ (Volume of a solid)

• Quartic: y ∝ x⁴, Example: L ∝ T⁴ (Stefan-Boltzmann)

A diagram illustrating Kepler's laws.

• General form y ∝ xⁿ, n is the power law index

 

The simplest four forms of proportionality — direct, inverse, square and inverse square — are the most common. The inverse square relation is particularly important in astronomy since gravity and light diminish with distance from their source according to an inverse square law. However, the index n in a power law relationship can have any value, positive or negative. Kepler's third law relates the period of a planet's orbit (P) to its distance from the Sun (a) with a form P² ∝ a³. Taking the square root of both sides, we see that P ∝ a^3/2, or P ∝ a^1.5. Or taking the cube root of both sides, we see that a ∝ P^⅔, or a ∝ P^0.67.